Tuesday, 16 August 2011
Monday, 15 August 2011
ENGINEERING MECHANICS: GEOMETRICAL ANALYSIS
Assignment No. : 01
| 1. | Solve the following equation for the two roots of x: x2 — 16 = 0 | |||||||
|
| 2. | Using the basic trigonomic functions, determine the length of side AB of the right triangle. | |||||||
|
| 4. | Solve the following equation for x, y, and z: x – y + z = –1 –x + y + z = –1 x + 2y – 2z = 5 | |||||||
|
| 5. | Using the basic trigonomic functions, determine the length of side AB of the right triangle. | |||||||
|
| 6. | Determine the angles | |||||||
|
| 7. | Determine the length of side AB if right angle ABC is similar to right angle A'B'C': | |||||||
|
| 9. | Solve the following equation for the two roots of x: — x2 + 5x = — 6 | |||||||
|
| 10. | Using the basic trigonomic functions, determine the length of side AB of the right triangle. | |||||||
|
Saturday, 30 July 2011
CENTROID OF A STRAIGHT & CURVED LINE:
©subhankar karmakar 2011
CENTROID OF A STRAIGHT LINE:
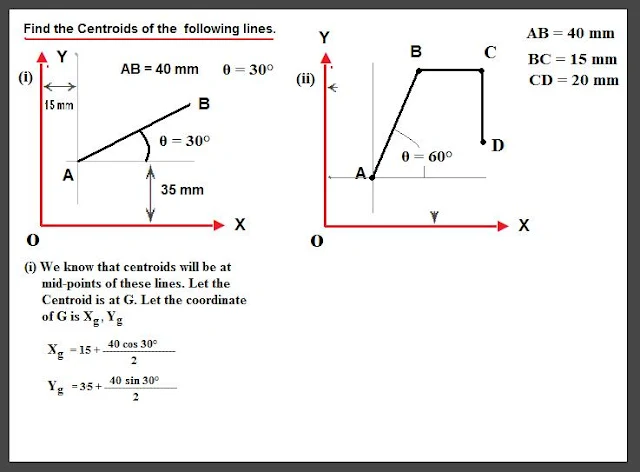
(a) Suppose we have straight lines of length (L) in a coordinate system. Let the lines are named as AB, BC and CA as shown in the figure. The centroids of these lines will at their mid-points viz. G1, G2, G3. The lines may be vertical, horizontal or inclined.
(b) For a curved line, a centroid G(Xg,Yg)can be defined by the equations,
Xg = (1/L)∫x.dL ------ (i)
Where dL = elemental length and L= total length of the line.
Yg = (1/L)∫y.dL ------ (ii)
Suppose we have a quarter circular arc in a co-ordinate system as shown in the figure. Total length of the arc AB = (πR)/2 . We take an arbitrarily small length of the arc CD and denote it as dL.
So, dL = Rdθ ------ (iii) [ as s=Rθ ]
Where R = Radius of the quarter circular arc. Let the co-ordinate of the point D be D(x,y) where
x = Rcosθ -----(iv) and y = Rsinθ -----(v)
x = Rcosθ -----(iv) and y = Rsinθ -----(v)
Hence Xg = (1/L)∫x.dL ;
here L = (πR)/2
x = Rcosθ
dL = Rdθ
Wednesday, 27 July 2011
TRUSS ANALYSIS: THEORY OF TRUSS:
Truss is a kind of framed structure made of entirely by rigid metallic rods joined by pin. The rods are called as Links or Linkages and the pins are called as joints. Their primary goal is to support the applied loads or we can say they are primarily load bearing structures. We often encounter trusses in our daily life as trusses are used to support roofs of various kinds of industrial sheds. Trusses are used as poles carrying high tension electricity.
LINK/ LINKAGES :
A link is a rigid rod which can bear any external load applied on it. A link can bear two types of forces.
COMPRESSIVE FORCES :
 When the external forces applied on the link or rod tries to decrease the length of the rod, then they are called as External Compressive Forces. A truss in equilibrium counters this compressive force by inducing an internal force, equal and opposite the externally applied force. The internal force thus induced balancing the external compressive force is named as Internal Compressive Forces. Generally Compressive Forces are considered as negative in truss analysis.
When the external forces applied on the link or rod tries to decrease the length of the rod, then they are called as External Compressive Forces. A truss in equilibrium counters this compressive force by inducing an internal force, equal and opposite the externally applied force. The internal force thus induced balancing the external compressive force is named as Internal Compressive Forces. Generally Compressive Forces are considered as negative in truss analysis. TENSILE FORCES :
When external loads applied on a link try to increase the length of the link, we call them External Tensile Loads. To neutral the tensile load applied on a link, an equal but opposite internal force is generated named as Internal Tensile forces. Tensile forces are generally considered as positive internal forces.
THE SIMPLEST TRUSS:
A triangular shaped truss made of three linkages and three joints is the simplest type of truss. As it is the simplest geometric shape where there is no change in shape with the application of forces at the joints if the length of rods/ linkages remain unchanged / constant.
MAXWELL'S TRUSS EQUATION:
To distinguish between "statically determinate structure" and "statically indeterminate structure" Maxwell formulated an equation involving the number of linkages (m) and number of joints (j).
The trusses which satisfies the equation,
m = 2j - 3
are statically determinate structures and named as "Perfect Trusses".
If m > 2j - 3, then the number of linkages are more than required, hence, called as "Redundant Trusses".
Where as if m < 2j - 3 for any truss, then the number of linkages are less than that of a perfect truss. These kinds of trusses are called as "Deficient Trusses".
ASSUMPTIONS CONSIDERED WHILE ANALYZING TRUSSES :
While analyzing trusses, to simplify the analysis we often consider certain assumptions. The purpose of these assumptions are the simplification of a complex problems. The assumptions are
(i) The links are perfectly rigid bodies, ie there occurs no change in the dimensions of the links.
(ii) The pin joints are perfectly smooth, ie there is no friction in the each and every joints.
(iii) The mass and weights of the links are so small compare to the magnitudes of the applied forces, that for truss analysis we shall neglect them. It means the links are massless as well as weightless.
(iv) The cross-sections and material of the links are uniform by nature.
(v) The external loads are only applied on a joint in the truss, whenever we shall place any external load, we must place it one of the joints in the truss.
(vi) Stress in each member is constant along its length.
The objective of analyzing the trusses is to determine the reactions and member forces. The methods used for carrying out the truss analysis with the equations of equilibrium and by considering only parts of the structure through analyzing its free body diagram to solve the unknowns.
Method of Joints
The first to analyze a truss by assuming all members are in tension reaction. A tension member is when a member experiences pull forces at both ends of the bar and usually denoted as positive (+ve) sign. When a member experiencing a push force at both ends, then the bar was said to be in compression mode and designated as negative (-ve) sign.
In the joints method, a virtual cut is made around a joint and the cut portion is isolated as a Free Body Diagram (FBD). Using the equilibrium equations of ∑ Fx = 0 and ∑ Fy = 0, the unknown member forces could be solve. It is assumed that all members are joined together in the form of an ideal pin, and that all forces are in tension (+ve) of reactions.
An imaginary section may be completely passed around a joint in the truss. The joint has become a free body in equilibrium under the forces applied to it. The equations ∑ H = 0 and ∑ V = 0 may be applied to the joint to determine the unknown forces in members meeting there. It is evident that no more than two unknowns can be determined at a joint with these two equations.
Figure 1: A simple truss model supported by pinned and roller support at its end. Each triangle has the same length, L and it is equilateral where degree of angle, θ is 60° on every angle. The support reactions, Ra and Rc can be determine by taking a point of moment either at point A or point C, whereas Ha = 0 (no other horizontal force).
Here are some simple guidelines for this method of truss analysis:
- Firstly draw the Free Body Diagram (FBD),
- Solve the reactions of the given structure,
- Select a joint with a minimum number of unknown (not more than 2) and analyze it with ∑ Fx = 0 and ∑ Fy = 0,
- Proceed to the rest of the joints and again concentrating on joints that have very minimal of unknowns,
- Check member forces at unused joints with ∑ Fx = 0 and ∑ Fy = 0,
- Tabulate the member forces whether it is in tension (+ve) or compression (-ve) reaction.
Figure 2: The figure showing 3 selected joints, at B, C, and E. The forces in each member can be determine from any joint or point. The best way to start by selecting the easiest joint like joint C where the reaction Rc is already obtained and with only 2 unknown, forces of FCB and FCD. Both can be evaluate with ∑ Fx = 0 and ∑ Fy = 0 rules. At joint E, there are 3 unknown, forces of FEA, FEB and FED, which may lead to more complex solution compare to 2 unknown values. For checking purposes, joint B is selected to shown that the equation of ∑ Fx is equal to ∑ Fy which leads to zero value, ∑ Fx = ∑ Fy = 0. Each value of the member’s condition should be indicate clearly as whether it is in tension (+ve) or in compression (-ve) state.
* (Trigonometric Functions:
Taking an angle between member x and z…
- Cos θ = x / z
- Sin θ = y / z
- Tan θ = y / x )
Method of Sections
The section method is an effective method when the forces in all members of a truss are being able to determine. Often we need to know the force in just one member with greatest force in it, and the method of section will yield the force in that particular member without the labor of working out the rest of the forces within the truss analysis.
If only a few member forces of a truss are needed, the quickest way to find these forces is by the method of sections. In this method, an imaginary cutting line called a section is drawn through a stable and determinate truss. Thus, a section subdivides the truss into two separate parts. Since the entire truss is in equilibrium, any part of it must also be in equilibrium. Either of the two parts of the truss can be considered and the three equations of equilibrium ∑ Fx = 0, ∑ Fy = 0, and ∑ M = 0 can be applied to solve for member forces.
Figure 3: Using the same model of simple truss, the details would be the same as previous figure with 2 different supports profile. Unlike the joint method, here we only interested in finding the value of forces for member BC, EC, and ED.
Few simple guidelines of section truss analysis:
- Pass a section through a maximum of 3 members of the truss, 1 of which is the desired member where it is dividing the truss into 2 completely separate parts,
- At 1 part of the truss, take moments about the point (at a joint) where the 2 members intersect and solve for the member force, using ∑ M = 0,
- Solve the other 2 unknowns by using the equilibrium equation for forces, using ∑ Fx = 0 and ∑ Fy = 0.
Figure 4: A virtual cut is introduce through the only required members which is along member BC, EC, and ED. Firstly, the support reactions of Ra and Rd should be determine. Again a good judgment is require to solve this problem where the easiest part would be consider either on the left hand side or the right hand side. Taking moment at joint E (virtual pint) on clockwise for the whole RHS part would be much easier compare to joint C (the LHS part). Then, either joint D or C can be consider as point of moment, or else using the joint method to find the member forces for FCB, FCE, and FDE. Note: Each value of the member’s condition should be indicate clearly as whether it is in tension (+ve) or in compression (-ve) state.
Tuesday, 26 July 2011
THE CONCEPT OF MOMENT:
Moment of force (or moment) is the tendency of a force to twist or rotate an object. This is an important, basic concept in engineering and physics. A moment is valued mathematically as the product of the force and the moment arm. The moment arm is the perpendicular distance from the point of rotation, to the line of action of the force. The moment may be thought of as a measure of the tendency of the force to cause rotation about an imaginary axis through a point. (Note: In mechanical and civil engineering, "moment" and "torque" have different meanings, while in physics they are synonyms.)
The moment of a force can be calculated about any point and not just the points in which the line of action of the force is perpendicular. Image A shows the components, the force F, and the moment arm, x when they are perpendicular to one another. When the force is not perpendicular to the point of interest, such as Point O in Images B and C, the magnitude of the Moment, M of a vector F about the point O is

In mechanical engineering (unlike physics), the terms "torque" and "moment" are not interchangeable. "Moment" is the general term for the tendency of one or more applied forces to rotate an object about an axis (the concept which in physics is called torque). "Torque" is a special case of this: If the applied force vectors add to zero (i.e., their "resultant" is zero), then the forces are called a "couple" and their moment is called a "torque".
For example, a rotational force down a shaft, such as a turning screw-driver, forms a couple, so the resulting moment is called a "torque". By contrast, a lateral force on a beam produces a moment (called a bending moment), but since the net force is nonzero, this bending moment is not called a "torque".

A Couple is a system of forces with a resultant (a.k.a. net, or sum) moment but no resultant force. Another term for a couple is a pure moment. Its effect is to create rotation without translation, or more generally without any acceleration of the centre of mass.
The resultant moment of a couple is called a torque. This is not to be confused with the term torque as it is used in physics, where it is merely a synonym of moment. Instead, torque is a special case of moment. Torque has special properties that moment does not have, in particular the property of being independent of reference point.
The simplest kind of couple consists of two equal and opposite forces whose lines of action do not coincide. This is called a "simple couple". The forces have a turning effect or moment called a torque about an axis which is normal to the plane of the forces. The SI unit for the torque of the couple is newton metre.
If the two forces are F and −F, then the magnitude of the torque is given by the following formula:
 , which is perpendicular to the plane containing the two forces. When d is taken as a vector between the points of action of the forces, then the couple is the cross product of d and F.
, which is perpendicular to the plane containing the two forces. When d is taken as a vector between the points of action of the forces, then the couple is the cross product of d and F.
Independence of reference point:
The moment of a force is only defined with respect to a certain point P (it is said to be the "moment about P"), and in general when P is changed, the moment changes. However, the moment (torque) of a couple is independent of the reference point P: Any point will give the same moment.In other words, a torque vector, unlike any other moment vector, is a "free vector". (This fact is called Varignon's Second Moment Theorem.)
The proof of this claim is as follows: Suppose there are a set of force vectors F1, F2, etc. that form a couple, with position vectors (about some origin P) r1, r2, etc., respectively. The moment about P is
The moment of a force can be calculated about any point and not just the points in which the line of action of the force is perpendicular. Image A shows the components, the force F, and the moment arm, x when they are perpendicular to one another. When the force is not perpendicular to the point of interest, such as Point O in Images B and C, the magnitude of the Moment, M of a vector F about the point O is
- where
is the vector from point O to the position where quantity F is applied.
- × represents the cross product of the vectors.
[In the figure a moment at Point O, when the components are perpendicular to the Point O. Image B and Image C illustrate the components of a Moment at Point O, when the components are not perpendicular to point O.]
In mechanical engineering (unlike physics), the terms "torque" and "moment" are not interchangeable. "Moment" is the general term for the tendency of one or more applied forces to rotate an object about an axis (the concept which in physics is called torque). "Torque" is a special case of this: If the applied force vectors add to zero (i.e., their "resultant" is zero), then the forces are called a "couple" and their moment is called a "torque".
For example, a rotational force down a shaft, such as a turning screw-driver, forms a couple, so the resulting moment is called a "torque". By contrast, a lateral force on a beam produces a moment (called a bending moment), but since the net force is nonzero, this bending moment is not called a "torque".
A particle is located at position r relative to its axis of rotation. When a force F is applied to the particle, only the perpendicular component F⊥ produces a torque. This torque τ = r × F has magnitude τ = |r| |F⊥| = |r| |F| sinθ and is directed outward from the page.
A Couple is a system of forces with a resultant (a.k.a. net, or sum) moment but no resultant force. Another term for a couple is a pure moment. Its effect is to create rotation without translation, or more generally without any acceleration of the centre of mass.
The resultant moment of a couple is called a torque. This is not to be confused with the term torque as it is used in physics, where it is merely a synonym of moment. Instead, torque is a special case of moment. Torque has special properties that moment does not have, in particular the property of being independent of reference point.
Simple Couple:
The simplest kind of couple consists of two equal and opposite forces whose lines of action do not coincide. This is called a "simple couple". The forces have a turning effect or moment called a torque about an axis which is normal to the plane of the forces. The SI unit for the torque of the couple is newton metre.
If the two forces are F and −F, then the magnitude of the torque is given by the following formula:
- τ is the torque
- F is the magnitude of one of the forces
- d is the perpendicular distance between the forces, sometimes called the arm of the couple
Independence of reference point:
The moment of a force is only defined with respect to a certain point P (it is said to be the "moment about P"), and in general when P is changed, the moment changes. However, the moment (torque) of a couple is independent of the reference point P: Any point will give the same moment.In other words, a torque vector, unlike any other moment vector, is a "free vector". (This fact is called Varignon's Second Moment Theorem.)
The proof of this claim is as follows: Suppose there are a set of force vectors F1, F2, etc. that form a couple, with position vectors (about some origin P) r1, r2, etc., respectively. The moment about P is
Wednesday, 20 July 2011
2D FORCE ANALYSIS : HOW TO FIND REACTIONS IN A CASE OF CONCURRENT FORCE SYSTEM ACTING ON A BODY IS IN EQUILIBRIUM
DEFINITION : CONCURRENT FORCE SYSTEM
If the lines of actions of all the forces in a force system pass through a common point, then the force system is called as Concurrent Force System. The equilibrium conditions for a concurrent force system is
If the lines of actions of all the forces in a force system pass through a common point, then the force system is called as Concurrent Force System. The equilibrium conditions for a concurrent force system is
ΣFx = 0 and ΣFy = 0
The steps to find out reactions when a coplanar concurrent force system acting on a body in equilibrium condition :
STEP 1 :
(i) Draw the diagram and identify all the contact points the body makes with other bodies including ground.
(ii) Draw a tangent at each contact point with the object. These tangents are called Contact Surfaces.
(iii) Draw a perpendicular to the contact surface at each and every contact points. These perpendiculars will be the directions of reactions at each and every contact point.
(iv) Find the angles made by the reactions with respect to horizontal with the help of Geometry.
STEP 2 :
(i) Draw the Free Body Diagram (FBD) that consists of the external forces acting on the object. (applied forces, forces of gravity and reactions all are external forces)
(ii) Assign reactions by symbols like R1, R2 ....... and resolve all the external forces along X-axis and Y-axis.
(iii) Now add all the horizontal component forces as ΣFx and put ΣFx = 0 ---- eqn (1)
and add all the vertical component forces as ΣFy and put ΣFy = 0 --------eqn (2)
(iv) Solving these two equations we shall get values of R1, R2.
Subscribe to:
Posts (Atom)