Showing posts with label EME-102. Show all posts
Showing posts with label EME-102. Show all posts
Thursday, 19 July 2012
QUESTIONS BANK 5 : FORCE AND FORCE SYSTEM
Labels:
2012-13,
B.Tech,
concurrent,
coplanar,
EME-102,
EME-202,
engineering,
ENGINEERING MECHANICS,
EQUILIBRIUM,
FORCE,
force system,
gbtu,
ghaziabad,
Lecture notes,
lectures on mechanics,
Mechanical,
mechanics,
mtu
QUESTIONS BANK 4 : FORCE AND FORCE SYSTEM
Labels:
2012-13,
B.Tech,
concurrent,
coplanar,
EME-102,
EME-202,
engineering,
ENGINEERING MECHANICS,
EQUILIBRIUM,
FORCE,
force system,
gbtu,
ghaziabad,
Lecture notes,
lectures on mechanics,
Mechanical,
mechanics,
mtu
QUESTIONS BANK 3 : FORCE AND FORCE SYSTEM
Labels:
2012-13,
B.Tech,
couple,
cross product,
EME-102,
EME-202,
ENGINEERING MECHANICS,
FORCE,
force system,
gbtu,
ghaziabad,
Lecture notes,
lectures on mechanics,
Mechanical,
mtu,
vector,
vector addition
Thursday, 12 July 2012
QUESTIONS BANK 2: FORCE AND FORCE SYSTEM
(I am going to publish a question bank for EME-102/EME-202 of 1st yr.
MTU; Greater Noida. Some pages from the book .......Subhankar Karmakar)
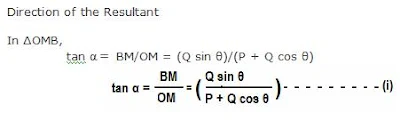
FOA = (P sin β)/ sin (α +β)
1) Explain the principle of Super-position.
Ans: The principle of superposition states
that “The effect of a force on a body does not change and remains same if we
add or subtract any system which is in equilibrium.”
In the fig 4 a, a force P is applied at
point A in a beam, where as in the fig 4 b, force P is applied at point A and a
force system in equilibrium which is added at point B. Principle of super
position says that both will produce the same effect.
2) What is “Force-Couple system?”
Ans: When a force is required to transfer
from a point A to point B, we can transfer the force directly without changing
its magnitude and direction but along with the moment of force about point B.
As a result of parallel transfer a system is obtained which is always a
combination of a force and a moment or couple. This system consists of a force
and a couple at a point is known as Force-Couple system.
In fig 5 a, a force P acts on a bar at point
A, now at point B we introduce a system of forces in equilibrium (fig 5 b),
hence according to principle of superposition there is no change in effect of
the original system. Now we can reduce the downward force P at point A and
upward force P at point B as a couple of magnitude Pxd at point B (fig 5 c).
3) What
do you understand by Equivalent force systems?
Ans: Two different force systems will be
equivalent if they can be reduced to the same force-couple system at a given
point. So, we can say that two force systems acting on the same rigid body will
be equivalent if the sums of forces or resultant and sums of the moments about
a point are equal.
4) What is orthogonal or perpendicular resolution of a
force?
Ans: The resolution of a force into
two components which are mutually perpendicular to each other along X-axis and
Y-axis is called orthogonal resolution of a force.
If a force F acts on an object at an angle θ with the
positive X-axis, then its component along X-axis is Fx = Fcosθ,
and that along Y-axis is Fy = Fsinθ
5) What
is oblique or non-perpendicular resolution of a force?
Ans: When a force is required to be resolved
in to two directions which are not perpendiculars to each other the resolution
is called oblique or Non-perpendicular resolution of a force.
FOA = (P sin β)/ sin (α +β)
FOB
= (P sin α)/ sin
(α +β)
Labels:
2012-13,
B.Tech,
concurrent,
coplanar,
EME-102,
EME-202,
engineering,
ENGINEERING MECHANICS,
EQUILIBRIUM,
FORCE,
force system,
gbtu,
ghaziabad,
Lecture notes,
lectures on mechanics,
Mechanical,
mechanics,
mtu
Wednesday, 11 July 2012
QUESTION BANK 1: FORCE AND FORCE SYSTEM
(I am going to publish a question bank for EME-102/EME-202 of 1st yr. MTU; Greater Noida. Some pages from the book .......Subhankar Karmakar)
QUESTION BANK: ENGINEERING MECHANICS
The fig 3 a shows a force F acting at a point of
application A and fig 3 b, the same force F acts along the same line of action
but at a different point of action at B and both are equivalent to each other.
QUESTION BANK: ENGINEERING MECHANICS
by Er. Subhankar Karmakar
Unit: 1 (Force System)
VERY SHORT QUESTIONS (2 marks):
1) What is force and force system?
Ans: A force is a physical quantity having magnitude as well as direction. Therefore, it is a vector quantity. It is defined as an "external agency" which produces or tends to produce or destroys or tends to destroy the motion when applied on a body.
Its unit is Newton (N) in S.I. systems and dyne in C.G.S. system.
When two or more forces act on a body or particle, it is called force system. Therefore, a force system is a collection of two or more forces.
2) What is static equilibrium? What are the different types
of static equilibrium?
Ans: A body is said to be in static equilibrium when there is
no change in position as well as no rotation exist on the body. So to be in
equilibrium process, there must not be any kind of motions ie there must not be
any kind of translational motion as well as rotational motion.
We
also know that to have a linear translational motion we need a net force acting
on the object towards the direction of motion, again to induce an any kind of
rotational motion, a net moment must exists acting on the body. Further it can
be said that any kind of complex motion can be resolved into a translational
motion coupled with a rotating motion.
“Therefore a body subjected to a force system would be at
rest if and only if the net force as well as the net moment on the body is
zero.”
There are three types of Static Equilibrium
1. Stable Equilibrium
2. Unstable Equilibrium
3. Neutral Equilibrium
3) What are the characteristics of a force?
Ans: A force has four (4) basic characteristics.
·
Magnitude: It is the value of
the force. It is represented by the length of the arrow that we use to
represent a force.
·
Direction: A force always
acts along a line, which is called as the “line of action”. The arrow head we
used to represent a force is the direction of that force.
·
Nature or Sense: The arrow head
also represent the nature of a force. A force may be a pull or a push. If a
force acts towards a particle it will be a push and if the force acts away from
a point it is pull.
·
Point of Application: It is the original
location of a point on a body where the force is acting.
4) What are the effects of a force acting on a body?
Whenever a force acts on a body or particle,
it may produce some external as well as internal effects or changes.
·
A force may change the state or position of a body by inducing
motion of the body. (External effect)
·
A force may change the size or shape of an object when applied
on it. It may deform the body thus inducing internal effects on the body.
·
A force may induce rotational motion into a body when applied at
a point other than its center of gravity.
·
A force can make a moving body into an equilibrium state at
rest.
5) What is composition and resolution of forces?
Ans: Composition
of forces: Composition or compounding is the procedure to find out single
resultant force of a force system
Resolution
of forces: Resolution is the procedure of splitting up a single force into
number of components without changing the effect of the same.
6) What is Resultant and Equilibrant?
Ans: Resultant: The resultant of a force system is
the Force which produces same effect as the combined forces of the force system
would do. So if we replace all components of the force by the resultant force,
then there will be no change in effect.
The Resultant of a force system is a vector addition of
all the components of the force system. The magnitude as well as direction of a
resultant can be measured through analytical method.
Equilibrant: Any concurrent set of forces, not
in equilibrium, can be put into a state of equilibrium by a single force. This
force is called the Equilibrant. It is equal in magnitude, opposite in sense
and co-linear with the resultant. When this force is added to the force system,
the sum of all of the forces is equal to zero.
7) Explain the principle of Transmissibility?
Ans: The principle of transmissibility states “the point
of application of a force can be transmitted anywhere along the line of action,
but within the body.”
Labels:
2012-13,
B.Tech,
concurrent,
coplanar,
EME-102,
EME-202,
engineering,
ENGINEERING MECHANICS,
EQUILIBRIUM,
FORCE,
force system,
gbtu,
ghaziabad,
Lecture notes,
lectures on mechanics,
Mechanical,
mechanics,
mtu
Sunday, 8 July 2012
NEW SYLLABUS FOR ENGINEERING MECHANICS: FIRST YEAR OF MTU FOR 2012-13
ENGINEERING MECHANICS
L T P
3 1 2
UNIT I
Two Dimensional Concurrent Force Systems: Basic concepts, Units, Force systems, Laws of motion, Moment and Couple, Vectors - Vector representation of forces and moments - Vector operations. Principle of Transmissibility of forces, Resultant of a force system, Equilibrium and Equations of equilibrium, Equilibrium conditions, Free body diagrams, Determination of reaction, Resultant of two dimensional concurrent forces, Applications of concurrent forces. 8
UNIT II
Two Dimensional Non-Concurrent Force Systems: Basic Concept, Varignon’s theorem, Transfer of a Force to Parallel Position, Distributed force system, Types of Supports and their Reactions, Converting force into couple and vise versa. 3
Friction: Introduction, Laws of Coulomb Friction, Equilibrium of bodies involving dry-friction, Belt friction, Ladder Friction, Screw jack 3
Structure: Plane truss, Perfect and Imperfect Truss, Assumption in the Truss Analysis, Analysis of Perfect Plane Trusses by the Method of Joints, Method of Section. 4
UNIT III
Centroid and Moment of Inertia: Centroid of plane, curve, area, volume and composite bodies,
Moment of inertia of plane area, Parallel Axes Theorem, Perpendicular axes theorems, Mass Moment of Inertia of Circular Ring, Disc, Cylinder, Sphere and Cone about their, Axis of Symmetry. Pappus-theorems, Polar moment of inertia. 8
UNIT IV
Kinematics of Rigid Body: Introduction, Plane Rectilinear Motion of Rigid Body, Plane Curvilinear Motion of Rigid Body, Velocity and Acceleration under Translation and Rotational Motion, Relative Velocity. 8
UNIT (V)
Kinetics of Rigid Body: Introduction, Force, Mass and Acceleration, Work and Energy, Impulse and Momentum, D’Alembert’s Principles and Dynamic Equilibrium, Friction in moving bodies. 8
Text books:
1. Engineering Mechanics Statics , J.L Meriam , Wiley
2. Engineering Mechanics Dynamics , J.L Meriam , Wiley
3. Engineering Mechanics – Statics & Dynamics by A Nelson, McGraw Hill
4. Engineering Mechanics : Statics and Dynamics, R. C. Hibbler
5. Mechanics of Solids by Abdul Mubeen, Pearson Education Asia.
6. Mechanics of Materials by E.P.Popov, Prentice Hall of India Private Limited.
ENGINEERING MECHANICS- LAB
(Any 10 experiments of the following or such experiments suitably designed)
1. Polygon law of Co-planer forces (concurrent)
2. Bell crank lever -Jib crane
3. Support reaction for beam
4. Collision of elastic bodies(Law of conservation of momentum
5. Moment of inertia of fly wheel.
6. Screw fiction by using screw jack
7. To study the slider-crank mechanism etc. of 2-stroke & 4-stroke I.C. Engine models.
8. Friction experiment(s) on inclined plane and/or on screw-jack.
9. Simple & compound gear-train experiment.
10. Worm & worm-wheel experiment for load lifting.
11. Belt-Pulley experiment. .
12. Experiment on Trusses.
13. Statics experiment on equilibrium
14. Dynamics experiment on momentum conservation
15. Dynamics experiment on collision for determining coefficient of restitution.
16. Simple/compound pendulum
Monday, 19 December 2011
LOADING IN BEAMS
BEAMS & CLASSIFICATION OF BEAMS
BEAM: A beam is a structure generally a horizontal structure on rigid supports and it carries mainly vertical loads. Therefore, beams are a kind of load bearing structures.
Depending upon the types of supports beams can be classified into different catagories.
CANTI-LEVER BEAMS:
A beam can be at stable equilibrium with a single fixed support at one end and the other end remains free, which is called as the free end while the other end is known as fixed end. This kind of beam is known as Canti lever beam. The fixed joint at the fixed end produces a horizontal, a vertical reactions and a reaction moment at the fixed end.
SIMPLE SUPPORTED BEAM:
A beam supported as just resting freely on the walls or columns at its both ends is known as simply supported beam.
There will be two vertically upward reactions at the ends of a simply supported beam. A simply supported beam can not resist any horizontal load component.
OVER HANGING BEAM:
A beam having its end portion or both the end portions extended in the form of a canti-lever beyond the support or supports is called as over hanging beam.
Above those beams are statically determinate. It means that those beams can be analysed applying the conditions of equilibrium. We can determine the values of the unknown reactions.
There are beams which can not be analysed applying the conditions of equilibrium of coplanar forces. These beams are also known as Statically indeterminate structures.
Those types of beams can be classified as,
Fixed beams and Continuous beams.
Fixed Beam: A beam having two fixed joints at the both ends is called fixed beam.
Continuous Beam: The beam which is at rest on more than two supports is called as continuous beam.
What are different types of supports?
There are four types of supports,
Depending upon the types of supports beams can be classified into different catagories.
CANTI-LEVER BEAMS:
A beam can be at stable equilibrium with a single fixed support at one end and the other end remains free, which is called as the free end while the other end is known as fixed end. This kind of beam is known as Canti lever beam. The fixed joint at the fixed end produces a horizontal, a vertical reactions and a reaction moment at the fixed end.
SIMPLE SUPPORTED BEAM:
A beam supported as just resting freely on the walls or columns at its both ends is known as simply supported beam.
There will be two vertically upward reactions at the ends of a simply supported beam. A simply supported beam can not resist any horizontal load component.
OVER HANGING BEAM:
A beam having its end portion or both the end portions extended in the form of a canti-lever beyond the support or supports is called as over hanging beam.
Above those beams are statically determinate. It means that those beams can be analysed applying the conditions of equilibrium. We can determine the values of the unknown reactions.
There are beams which can not be analysed applying the conditions of equilibrium of coplanar forces. These beams are also known as Statically indeterminate structures.
Those types of beams can be classified as,
Fixed beams and Continuous beams.
Fixed Beam: A beam having two fixed joints at the both ends is called fixed beam.
Continuous Beam: The beam which is at rest on more than two supports is called as continuous beam.
What are different types of supports?
There are four types of supports,
- (i) Simple Supports,
- (ii) Roller Supports,
- (iii) Hinged Supports
- (iv) Fixed Supports.
Thursday, 1 December 2011
SOLUTION OF EME-102; CENTROID
HOW TO FIND THE CENTROID OF A COMPOSITE AREA
(a composite area consists of several straight or curved lines.)
(i) Draw the figure in a coordinate system. Draw the dimensions too. Every dimensions will be measured with respect to origin of the coordinate system
(ii) Divide the composite area into several parts of basic geometric areas. Lebel them as part-1, part-2, part-3, .......part-n. Let the corresponding areas are A1, A2, A3, .... An. Let the centroids are G1(X1,Y1), G2(X2,Y2), G3(X3,Y3), ...... Gn(Xn,Yn).
(iii) Let the centroid of the composite area be G(Xg,Yg). Hence,
Xg = (A1X1 + A2X2 +A3X3)/(A1 + A2 + A3)
Yg = (A1Y1 + A2Y2 +A3Y3)/(A1 + A2 + A3)
(a composite area consists of several straight or curved lines.)
(i) Draw the figure in a coordinate system. Draw the dimensions too. Every dimensions will be measured with respect to origin of the coordinate system
(ii) Divide the composite area into several parts of basic geometric areas. Lebel them as part-1, part-2, part-3, .......part-n. Let the corresponding areas are A1, A2, A3, .... An. Let the centroids are G1(X1,Y1), G2(X2,Y2), G3(X3,Y3), ...... Gn(Xn,Yn).
(iii) Let the centroid of the composite area be G(Xg,Yg). Hence,
Xg = (A1X1 + A2X2 +A3X3)/(A1 + A2 + A3)
Yg = (A1Y1 + A2Y2 +A3Y3)/(A1 + A2 + A3)
(a) Suppose we have certain area of magnitude (A) in a coordinate system. The centroid of the area will be at its mid-point. A centroid is denoted by G.
In the figure we have a complex geometrical area composed of three basic geometrical areas. A rectangle, a semi circle and a isosceles triangle. Let us denote the centroids as G1, G2, G3 for the given areas in the figure.
We shall have to find the Centroid of the entire area composed of A1, A2, A3.
At first, the composite line is divided into three parts.
In the figure we have a complex geometrical area composed of three basic geometrical areas. A rectangle, a semi circle and a isosceles triangle. Let us denote the centroids as G1, G2, G3 for the given areas in the figure.
We shall have to find the Centroid of the entire area composed of A1, A2, A3.
At first, the composite line is divided into three parts.
Part -1 : The semi-circle : Let the centroid of the area A1 be G1(X1,Y1)
Area, A1 = (π/2)x(25)² mm² = 981.74 mm²
X1 = { 25 - (4x25)/(3xπ)} mm = 14.39 mm
Y1 = 25 mm
Part -2 : The Rectangle : Let the centroid of the A2 be G2(X2,Y2)
Area, A2 = 100 x 50 mm² = 5000 mm²
X2 = 25 + (100/2) = 75 mm
Y2 = 25 mm
Part -3 : The Triangle : Let the centroid of the area Area, A3 be G3(X3,Y3)
Area, A3 = (1/2) x 50 x 50 mm² = 1250 mm²
X3 = 25 + 50 + 25 = 100 mm
Y3 = 50 + (50/3) = 66.67 mm
If the centroid of the composite line be G (Xg,Yg)
X1 = { 25 - (4x25)/(3xπ)} mm = 14.39 mm
Y1 = 25 mm
Part -2 : The Rectangle : Let the centroid of the A2 be G2(X2,Y2)
Area, A2 = 100 x 50 mm² = 5000 mm²
X2 = 25 + (100/2) = 75 mm
Y2 = 25 mm
Part -3 : The Triangle : Let the centroid of the area Area, A3 be G3(X3,Y3)
Area, A3 = (1/2) x 50 x 50 mm² = 1250 mm²
X3 = 25 + 50 + 25 = 100 mm
Y3 = 50 + (50/3) = 66.67 mm
If the centroid of the composite line be G (Xg,Yg)
Xg = (∑AiXi)/(∑Ai)
= (A1X1 + A2X2 +A3X3)/(A1 + A2 + A3)
= (981.74 x 14.39 + 5000 x 75 + 1250 x 100)/( 981.74 + 5000 + 1250)
= 71.09
Yg = (∑AiYi)/(∑Ai)
= (A1Y1 + A2Y2 +A3Y3)/(A1 + A2 + A3)
= (981.74 x 25 + 5000 x 25 + 1250 x 66.67)/ ( 981.74 + 5000 + 1250)
= 32.20
SOLUTION OF EME-102; EQUILIBRIUM OF FORCES
EQUILIBRIUM OF FORCES IN 2D
A light string ABCDE whose extremity A is fixed, has weights W1 & W2 attached to it at B & C. It passes round a small smooth pulley at D carrying a weight of 300 N at the free end E as shown in figure. If in the equilibrium position, BC is horizontal and AB & CD make 150° and 120° with BC, find (i) Tensions in the strings and (ii) magnitudes of W1 & W2
Although ABCDE is a single string/rope but still the tensions in the string/rope will be different at different segments like in the segment AB the tensions will be T1 , but in BC segment it will be different as the weight is attached at a fixed point (point B) on the string, hence it will be T2 here and in CD it will be T3 there. As at point D the string is not attached rather passes over a smooth pulley hence the tension in DE and CD will be same ie. T3 again.
.
Although ABCDE is a single string/rope but still the tensions in the string/rope will be different at different segments like in the segment AB the tensions will be T1 , but in BC segment it will be different as the weight is attached at a fixed point (point B) on the string, hence it will be T2 here and in CD it will be T3 there. As at point D the string is not attached rather passes over a smooth pulley hence the tension in DE and CD will be same ie. T3 again.
.
To solve for equilibrium of forces in 2D, follow these steps:
1. Draw a free body diagram: Draw a diagram of the object in question and identify all the forces acting on it. This will help you visualize the problem and identify any unknown forces or angles.
2. Break forces into components: Resolve all forces into their x- and y-components. This is done by using trigonometry to determine the horizontal and vertical components of each force.
3. Apply Newton's second law: For the object to be in equilibrium, the sum of the forces in the x-direction and the sum of the forces in the y-direction must be equal to zero. This gives you two equations to solve simultaneously.
4. Solve the equations: Solve the equations for the unknown forces or angles by using algebraic methods. This will give you the values of the unknown forces or angles required for the object to be in equilibrium.
5. Check for consistency: Check that the forces and angles you have calculated are consistent with the problem and the free body diagram. For example, make sure that the direction of the forces makes sense and that the angles are reasonable.
6. Interpret the results: Interpret the results and explain what they mean in the context of the problem. This might involve calculating the tension in a rope, the force required to lift an object, or the angle required for an object to remain stationary.
Monday, 28 November 2011
QUESTION BANKS: Analyse the following Trusses:
Analyse the following Trusses:
(1) A cantilever truss has been as shown in the figure. Find the value of W which will produces a force of magnitudes 15 kN in the member AB.
(2) A cantilever truss is loaded as shown in the figure. Find the nature and magnitudes of the forces in each link.
(3) A cantilever truss has been as shown in the figure. Find the value of W which will produces a force of magnitudes 15 kN in the member AB.
(4) A truss has been loaded as shown in the figure. Find the nature and the magnitudes of the forces in the links BC, CH and GH by the methods of sections.
(6) A truss has been loaded as shown in the figure. Find the forces in each member and tabulate them by any methods.
compiled by Subhankar Karmakar
more content: click the following links for more questions.
THEORETICAL QUESTIONS ON SIMPLE TRUSSES part-3
QUESTION BANK : ENGINEERING MECHANICS PART-2
QUESTION BANK : ENGINEERING MECHANICS
THEORETICAL QUESTIONS ON SIMPLE TRUSSES
SHORT QUESTIONS: TOPIC - TRUSS ANALYSIS
1) What is a truss? Classify them with proper diagrams.
2) State the differences between a perfect truss and an imperfect truss.
3) Distinguish between a deficient truss and a redundant truss.
4) Write the Maxwell’s Truss Equation.
5) What are the assumptions made, while finding out the forces in the various members of a truss?
6) What are the differences between a simply supported truss and a cantilever truss? Discuss the method of finding out reactions in both the cases.
Analyse the following Trusses:
(1) Analyse the Truss by the method of Joints.
(2) Find the internal forces on the links 1, 2 and 3 by the method of Sections.
(3) Determine the magnitude and the nature of the forces in the members BC, GC and GF of the given truss.
(4) A truss of span 10 m is loaded as shown in the figure. Find the forces in all the links by any method.
compiled by Subhankar Karmakar
compiled by Subhankar Karmakar
PARALLEL AXIS THEOREM AND IT'S USES IN MOI
Moment Of Inertia of an Area.
MOI or MOMENTS OF INERTIA is a physical quantity which represents the inertia or resistances shown by the body against the tendency to rotate under the action external forces on the body. It is a rotational axis dependent function as its magnitude depends upon our selection of rotational axis. Although for any axis, we can derive the expression for MOI with the help of calculus, but still it is a cumbersome process.Now suppose we take a different issue. We know MOI of an area about its centroidal axis is easily be obtained by integral calculus, but can we find a general formula by which we can calculate MOI of an area about any axis if we know its CENTROIDAL MOI.
We shall here find that we can indeed derive an expression by which MOI of any area (A) can be calculated about any Axis, if we know its centroidal MOI and the distance of the axis from it's Centroid G.
If IGX be the centroidal moment of inertia of an area (A) about X axis, then we can calculate MOI of the Area about a parallel axis (here X axis passing through the point P) at a distance Ŷ-Y'=Y from the centroid if we know the value of IGX and Y, then IPX will be
IPX = IGX + A.Y2 where Y=Ŷ-Y'
IXX = IOX = IGX + A.Ŷ2
Where IXX is the moment of inertia of the area about the co-ordinate axis parallel to X axis and passing through origin O, hence we can say,
IXX = IOX
IMPORTANT: The notation of Moment of Inertia
MOI of an area about an axis passing through a point B will be written as IBX
Q: Find the Centroidal Moment of Inertia of the figure given above. Each small division represents 50 mm.
To find out Centroidal MOI
Wednesday, 24 August 2011
CENTROIDS OF LINES
 If we take a straight line of length (L), then its midpoint will be at a distance (L/2) from either end of the line. Let us denote the centroid as the point G(Xg,Yg).
If we take a straight line of length (L), then its midpoint will be at a distance (L/2) from either end of the line. Let us denote the centroid as the point G(Xg,Yg). Suppose we have a straight line AB of length (L) that makes an angle θ with X axis. Let the coordinate of point A is (Xo,Yo). Let the centroid be at G(Xg,Yg), then
Xg = Xo + (Lcos θ)/2
Yg = Yo + (Lsin θ)/2
Xg = X1 - (Lcos θ)/2
Yg = Y1 - (Lsin θ)/2
For Horizontal lines θ = 0° and for Vertical lines θ = 90°
CENTROID OF A CURVED LINE
The steps to derive the centroid of a quarter circular arc of radius R.
Centroid of a curved line can be derived with the help of calculus.
i) Draw the figure in a X-Y coordinate system. Let the curved line has been represented by a function θ
ii) Take an arbitrary point P(X,Y) on the curve. Join the line OP, where O is the origin of the coordinate system. Let OP has a length L and makes an angle (θ) with X axis. Therefore, we can write
X = Rcosθ ----- (a)
Y = Rsinθ ----- (b)
iii) Let another point Q, such that PQ = dL where dL is very very small. Let the angle subtended by (dL) be (dθ). So we can write
Y = Rsinθ ----- (b)
iii) Let another point Q, such that PQ = dL where dL is very very small. Let the angle subtended by (dL) be (dθ). So we can write
dL = Rdθ ------ (c)
Xg = (1/L) ∫(XdL)
= (1/L) ∫ Rcosθ.Rdθ
= (1/L).R² ∫ sinθ.dθ ------- (d)
Yg = (1/L) ∫ YdL
= (1/L) ∫ Rsinθ.Rdθ
= (1/L).R² ∫ sinθ.dθ -------- (e)
Xg = (1/L) ∫(XdL)
= (1/L) ∫ Rcosθ.Rdθ
= (1/L).R² ∫ sinθ.dθ ------- (d)
Yg = (1/L) ∫ YdL
= (1/L) ∫ Rsinθ.Rdθ
= (1/L).R² ∫ sinθ.dθ -------- (e)
CENTROID OF A QUARTER CIRCULAR ARC OF RADIUS R
Suppose we have a quarter circular arc in a co-ordinate system as shown in the figure. Total length of the arc AB = (πR)/2 . We take an arbitrarily small length of the arc CD and denote it as dL.
dL = Rdθ ------ (iii) [ as s=Rθ ]
where R = Radius of the quarter circular arc.
Let the co-ordinate of the point D be D(x,y) where
X = Rcosθ -----(iv) and
Y = Rsinθ -----(v)
Hence Xg = (1/L)∫x.dL ; here L = (πR)/2 ;
X = Rcosθ
dL = Rdθ
Xg = (2/πR) 0∫π/2Rcosθ.Rdθ
= (2/πR) R2 0∫π/2cosθ.dθ
= 2R/π
Yg = (2/πR) 0∫π/2Rsinθ.Rdθ
= (2/πR) R2 0∫π/2sinθ.dθ
= 2R/π
Hence, for a quarter circular arc of radius R will be G(2R/π,2R/π)
CENTROID OF A COMPOSITE LINE
In the figure, a composite line A-B-C-D is made of three straight lines AB, BC, CD as shown in the figure.So, a composite line is consisted of several straight or curved lines.
Let a composite line is made of n number of lines, which may straight or curved lines.
STEP-ONE:
Draw the composite line and break it into n number of parts. Let the lengths of these lines are L1, L2, L3 ........ Ln. Let the centroids of these lines are G1(X1,Y1),G2(X2,Y2), G3(X3,Y3) ........ Gn(Xn,Yn).
Calculate length (Li), and coordinates (Xi,Yi) for each and every parts.
Let a composite line is made of n number of lines, which may straight or curved lines.
STEP-ONE:
Draw the composite line and break it into n number of parts. Let the lengths of these lines are L1, L2, L3 ........ Ln. Let the centroids of these lines are G1(X1,Y1),G2(X2,Y2), G3(X3,Y3) ........ Gn(Xn,Yn).
Calculate length (Li), and coordinates (Xi,Yi) for each and every parts.
Now, if the centroid of the composite line be G(Xg,Yg)
Xg = (∑LiXi)/(∑Li)
=> (L1X1 + L2X2 + L3X3)/(L1 + L2 + L3)
Yg = (∑LiYi)/(∑Li)
=> (L1Y1 + L2Y2 + L3Y3)/(L1 + L2 + L3)
Labels:
B.Tech,
center of gravity,
centroid,
composite,
curved,
EME-102,
eme-201,
engineering,
gbtu,
lectures on mechanics,
line,
Mechanical,
mtu,
physics,
statics,
straight,
subhankar,
UPTU,
WBTU
Subscribe to:
Posts (Atom)



































.jpg)


