THEORETICAL QUESTIONS ON SIMPLE TRUSSES

SHORT QUESTIONS: TOPIC - TRUSS ANALYSIS

1)      What is a truss? Classify them with proper diagrams.

2)      State the differences between a perfect truss and an imperfect truss.

3)      Distinguish between a deficient truss and a redundant truss.

4)      Write the Maxwell’s Truss Equation.

5)      What are the assumptions made, while finding out the forces in the various members of a truss?

6)      What are the differences between a simply supported truss and a cantilever truss? Discuss the method of finding out reactions in both the cases.

Analyse the following Trusses:

 

(1)      Analyse the Truss by the method of Joints.

(2) Find the internal forces on the links 1, 2 and 3 by the method of Sections.

(3) Determine the magnitude and the nature of the forces in the members BC, GC and GF of the given truss.

(4)   A truss of span 10 m is loaded as shown in the figure. Find the forces in all the links by any method.






compiled by Subhankar Karmakar 

PARALLEL AXIS THEOREM AND IT'S USES IN MOI

Moment Of Inertia of an Area.

download pdf file of solved numerical

MOI or MOMENTS OF INERTIA is a physical quantity which represents the inertia or resistances shown by the body against the tendency to rotate under the action external forces on the body. It is a rotational axis dependent function as its magnitude depends upon our selection of rotational axis. Although for any axis, we can derive the expression for MOI with the help of calculus, but still it is a cumbersome process.


Now suppose we take a different issue. We know MOI of an area about its centroidal axis is easily be obtained by integral calculus, but can we find a general formula by which we can calculate MOI of an area about any axis if we know its CENTROIDAL MOI.

We shall here find that we can indeed derive an expression by which MOI of any area (A) can be calculated about any Axis, if we know its centroidal MOI and the distance of the axis from it's Centroid G.


If I_GX be the centroidal moment of inertia of an area (A) about X axis, then we can calculate MOI of the Area about a parallel axis (here X axis passing through the point P) at a distance Ŷ-Y'=Y from the centroid if we know the value of I_GX and Y, then I_PX will be
I_PX = I_GX + A.Y² where Y=Ŷ-Y'


I_XX = I_OX = I_GX + A.Ŷ²

<sup>Where I_XX is the moment of inertia of the area about the co-ordinate axis parallel to X axis and passing through origin O, hence we can say,

I_XX = I_OX

 IMPORTANT: The notation of Moment of Inertia

MOI of an area about an axis passing through a point B will be written as I_BX</sup>

Q: Find the Centroidal Moment of Inertia of the figure given above. Each small division represents 50 mm.

To find out Centroidal MOI

TEST PAPER: EME-102: ENGINEERING MECHANICS

TEST PAPER: EME- EME-303: THERMODYNAMICS

Section A:

      (1)           Attempt All The Questions:                                             5x2 = 10

a)     Define system & surroundings.

b)    What is heat pump & refrigerator?

c)     What is availability?

d)    What is Entropy?

e)  What is triple point of water?

Section B:

    (2)          Attempt any three questions                                                          3x5 = 15

    

(a)    Distinguish between microscopic & macroscopic approaches of thermodynamics.

(b)   What are the limitations of First law of thermodynamics? Explain the statements of Second law of thermodynamics.

(c)    2 kg of a gas at 10 bar expands adiabatically and reversibly till the pressure drops to 5 bar. During the process 120 kJ of non-flow work is done by the system, and the temperature falls from 377° centigrade to 257°C. Calculate the value of the index of expansion and the characteristics gas constants.

Let the equation of expansion be P1-γ.Tγ = constant Hence, P1(1-γ)T1γ = P2(1-γ)T2γ

(d)   Derive the Tds equations.

(e)    Steam at a pressure of 4 bar absolute and having dryness fraction of 0.75 is heated at constant volume to a pressure of 5 bar absolute. Find the final condition of the steam and the heat absorbed by 1 kg of steam.

Section C:

Attempt part (a) or part (b) of the following questions                           5x5=25

(3) (a) Explain thermodynamic equilibrium and quasi-static process.

     (b) A steam turbine developing 110 kW is supplied steam at 17.5 bar with an internal energy of 2600 kJ/min and specific volume of 0.155 m³/kg and velocity of 100 m/s. exhaust from turbine is at 0.1 bar with internal energy of 2093 kJ/min and sp. Volume = 15.5 m³/kg and velocity of 275 m/s. heat loss from the steam turbine 37.6 kJ/kg neglecting potential energy changes, determine steam flow rate in kg/hr.

(4)(a) Prove the equivalence of Kelvin-Planck statement & Clausius statement.

     (b) A reversible engine takes 2400 kJ/min from a reservoir at 750 K develops 400 kJ/min of work during the cycle. The engine rejects heat to two reservoirs at 650 K & 550 K. Find the heat rejected to each sink.

(5)(a) Explain the principle of entropy increase.

    (b) Explain the Gibbs Function & Gibbs free energy

           

(6)(a) Distinguish between Universal gas constant and characteristics gas constant with proper example.

    (b) Explain the causes of internal and external irreversibility.

(7)(a) A gas having a moleculer mass of 28 occupies 0.13 m³ at a pressure of 1.5 bar and a temperature 21°C. Find the mass of gas and the volume as well as the density at 0°C and 1 bar pressure.

(b)    One kg of an ideal gas is heated from 18.3°C to 93.4°C. Assuming R=287 J/kg-K and

 γ = 1.18 for the gas. Find out (i) specific heats, (ii) change in internal energy and

(iii) change in enthalpy

T H E R M O D Y N A M I C S

 T H E R M O D Y N A M I C S


(1) Explain briefly what you understand about Microscopic and Macroscopic approaches to study Thermodynamics. 

or

Differentiate between them. Also state which approaches is considered in studying Engineering thermodynamics.


ANSWER: There are two approaches to study thermodynamic problem. They are known as

• (i) Microscopic approach and
• (ii) Macroscopic approach.

(i) If we try to analyse a system by considering it as comprising of discrete particles which are its atoms and molecules, we say that the approach is microscopic here. Here mass is regarded as a macro object which are composed of billions of billions microscopic particles known as atoms and molecules.


Where as when we analyse a system by its gross or time averaged effects of molecules we say the approach is macroscopic. Here matter is assumed to be continuous not discrete. It is regarded as a continuum, just like a physical field is taken as continuum.


(ii) As no. of molecules are very large hence its not possible to study individual molecules, hence the analysis in microscopic approach is done by statistical methods with the help of the theory of probability and the concept is known as Statistical Mechanics.


Where as in macroscopic approach the analysis is done on the basis classical or Newtonian Mechanics.


(iii) In Microscopic approach Statistical Mechanics and different probability distribution theories like Maxwell's velocity distribution theory have been employed.


Where as in Macroscopic approach is based on classical mechanics and calculus.




(iv) In Microscopic approach the value of the system parameters are indirectly calculated as most of them cant be directly measured.


Where as in macroscopic approach most of properties are not only measurable but also sensible too.






(2) What do you understand by the term Temperature? What is Thermal Equilibrium?


Here I want to write my view points about the term "equilibrium" with it's precise definition as an physical real events.


We all know that although we comprehend matter as a continuous distribution of masses that means that we can take infinitesimally and arbitrarily small volume of mass, but in reality, matter is composed of tiny particles called molecules. These molecules in gases are almost free of intermolecular forces, and always move randomly which is named as Brownian Motion. Gas molecules posses kinetic energy, so whenever they collide with the wall of the container within which the gas has been kept. Every collision is responsible for the momentum transfer to the wall which the wall resisted due to it's elastic properties. The change in momentum produces a thrust to the wall and we call it the pressure of the gas which means total force per unit surface area of the wall of the container. So, gross kinetic energy of the molecules due to their random Brownian motion has two effects on the wall of the container, one is due to the thrust on the wall named as Pressure of the enclosed gas. And the average Kinetic Energy of a molecules is the basis of stored energy of the gas molecules and we perceive it as temperature.


     When a body at certain temperature, T₁ is kept in contact with another body having a different temperature T₂, it means that there exists a difference in average kinetic energy of the molecules between the bodies. 




(3) Explain the term Thermodynamic Equilibrium. Explain the conditions of Thermodynamic Equilibrium. Also explain the conditions of Thermodynamic Equilibrium.                            (5) 

Ans: Equilibrium is a state or condition of a system, when there is no change in the value of properties with respect to time. In equilibrium condition, there exists no driving force inside the system and absence of driving force ensures that there is no change in the properties of the system. Basically, changes occur due to the existence of either (a) a temperature gradient, (b) a pressure gradient or (c) chemical potential in the system or between system and surroundings.  

Based on these equilibrium conditions are of three types. 

1. Thermal Equilibrium
2. Mechanical Equilibrium
3. Chemical Equilibrium 

(i) Thermal Equilibrium : If there is not any temperature difference between a system and its surroundings, there will not be any kind of heat exchange between the system and the surroundings. This state or condition of a system is known as Thermal Equilibrium of the system.

(ii) Mechanical Equilibrium: If there doesn't exist any pressure difference between a system and its surroundings, then the system is in Mechanical Equilibrium and  it tells that there will not be any work interactions between a system and its surroundings.

(iii) Chemical Equilibrium: If in a system that contains multi-components working fluid/substance and if there is not any chemical potential between them, then there will not be any chemical reaction inside a system and this condition is called as chemical equilibrium.

When a system is in thermal, mechanical and chemical equilibrium, then the system is called is in Thermodynamic equilibrium.






(4) Explain the statements of Second law of thermodynamics.

Answer: Kelvin Planck and Clausius statements of the second law of Thermodynamics.

The second law of thermodynamics can also be stated using Clausius, Kelvin and Planck statements also. Each statement is based on an irreversible process. The Clausius and the Kelvin and Planck statements of the second law of Thermodynamics are given below:


Clausius statement:
 
Clausius statement states “it is impossible for a self acting machine working in a cyclic process without any external force, to transfer heat from a body at a lower temperature to a body at a higher temperature. It considers transformation of heat between two heat reservoirs.


Kelvin – Planck statement:
 
Kelvin – Planck statement states “it is impossible to construct an engine, which is operating in a cycle produces no other effect except to external heat from a single reservoir and do equivalent amount of work.
It considers the transformation of heat into work.


Equivalence of Clausius statement to the Kelvin – Planck statement
 
Consider a reservoir having temperature T₁ and another reservoir at temperature T₂. The temperature T₁ is higher than the temperature T₂. Consider a heat pump which requires no work and transfers an amount of Q₂ from low temperature to a higher temperature reservoir, which is violating the Clausius statement. Consider an amount of heat Q₁ (greater than Q₂) be transferred from higher temperature reservoir to a heat engine which develops a net work, W = Q₁ – Q₂ and rejects Q₂ to the low temperature reservoir.
Since there is no heat interaction with the low temperature, it can be eliminated. The combined system of the heat engine and heat pump acts then like a heat engine exchanging heat with a single reservoir, which violates the Kelvin – Planck statement.

 

 

(5) Explain the concepts of continuum with the help of density.explain whether we can term density as an intensive properties of the system,justify your answer.


Ans: "Continuum" is a concept.

(6)What is a thermodynamic process? Explain the differences between reversible and irreversible process? What are the common causes of irreversibility.


(7)"....heat and work done are actually different forms of energy...and we termed them as energy in transition..." justify the statement.

or

Compare Heat Transfer with Work transfer...also explain why they are termed as path function? Differentiate between path and point function of a thermodynamic system.



(8) Discuss the importance of zeroth law. discuss its role in temperature measurement


(9) What is internal energy? Prove that internal energy is a property of the system. 


(10) What is the difference between flow work and pdV or displacement work. Also explain the term enthalpy.


(11) Classify thermodynamic system with example.discuss each of them briefly.


(12) What is SSSF energy equation? explain it and derive the equation.












First Law of Thermodynamics:

Statement: When a closed system executes a complete cycle the sum of heat interactions is equal to the sum of work interactions.
Mathematically, ΣQ=Σ W
The summations being over the entire cycle.

QUESTION BANK : ENGINEERING MECHANICS PART-2

TOPICS: NUMERICALS ON FORCE SYSTEM- UNIT-1

5) A bar of AB 12 m long rests in horizontal position on two smooth planes as shown in the figure. Find the distance X at which 100 kN is to be placed to keep the bar in equilibrium.

 

6) A light string ABCDE whose extremity A is fixed, has weights W₁ & W₂ attached to it at B & C. It passes round a small smooth pulley at D carrying a weight of 300 N at the free end E as shown in figure. If in the equilibrium position, BC is horizontal and AB & CD make 150° and 120° with BC, find (i) Tensions in the strings and (ii) magnitudes of W₁ & W₂  

 

7) Find reactions at all the contact points if weight of P is 200 N & diameter is 100 mm, where as weight of Q is 500 N and diameter is 180 mm.

 

8) Determine the force P required to begin rolling the uniform cylinder of mass (m) over the obstacle of height (h) as shown in the figure.  

 

9) A roller of weight 500 N has a radius of 120 mm and is pulled over a step of height 60 mm by a horizontal force P. Find magnitudes of P to just start the roller over the step.

 

10) Two identical rollers each of weight 100 N are supported by an inclined plane of 30° with horizontal and a vertical wall as shown in the figure. Find all the reactions at each contact point.

 

11) A smooth cylinder of radius 500 mm rests on a horizontal plane and is kept from rolling by a rope OA of 1000 mm length. A bar AB of length 1500 mm and weight 1000 N is hinged at point A and placed against the cylinder of negligible weight. Determine the tension in the rope.

 

12)      A flat belt connects pulley B, which drives a pulley A; attached to an electric motor. μ_s =  0.25 and μ_k = 0.2 between both the pulleys and the belt. If maximum allowable tension in the belt is 600 N, determine the largest torque which can be exerted by belt on pulley B.

      

13)       Two blocks of mass MA & MB are kept at equilibrium as shown in the figure. The friction between the block B & the floor is 0.35 and between the blocks is 0.3, then find the minimum force P to just move the block B.

QUESTION BANK : ENGINEERING MECHANICS

Compiled by Er. Subhankar Karmakar

Unit: 1 (Force System)

VERY SHORT QUESTIONS (2 marks):

1)      What is force & force system?

2)      What is equilibrium? What are the conditions of equilibrium?

3)      Distinguish between coplanar concurrent & coplanar non-concurrent forces.

4)      State & explain the law of transmissibility of forces.

5)      What is moment? What is couple?

6)      State and explain the Varignon’s theorem of moment.

7)      What are the characteristics of couple?

8)      What are parallel forces?

9)      State Lami’s theorem of equilibrium of forces?

10)  Where do we can apply Lami’s theorem?

11)  Explain the terms Resultant & equilibrant?

12)  What is friction? On what factors frictional force depends?

13)  Explain the term Ladder Friction?

14)  What is angle of friction, angle of repose, co-efficient of friction?

15)  Explain the concept of Limiting friction.

16)  Explain the cone of friction. Also discuss it’s physical significance?

17)  State Coulomb’s law of dry friction?

18)  What is a belt drive? Explain the term angle of contact or lap angle.

19)  Explain the term Torque, Couple and Moment?

20)  Distinguish between static and dynamic friction.

BROAD QUESTIONS (5 marks / 10 marks)

1)      Classify Force systems with proper examples.

2)      State & prove Lami’s theorem.

3)      State & prove Varignon’s theorem of moment.

4)      What is the resultant of a force system? How can we determine the resultant?

5)      What are support reactions? Describe different types of loading.

6)      What is distributed loading? Explain UDL & UVL with examples.

7)      What is a fixed supports? Explain the term reaction moment?

8)      State & explain the principle of superposition.

9)      Show that a force at a point can be reduced to a force-couple system at another point on the same plane.

10)  What is the meaning of Equivalent System?

11)  What is Free Body Diagram or FBD?

12)  Prove that for a belt drive, (T₁/T₂) = e^μθ

 

NUMERICALS: (10 marks)

(1) Two rigid rod BA & CA are joined together at point A as shown in the figure. Now a weight of 200 kN is placed at point A. Find the reaction forces in the rod AB & AC.

    [ R_AC = 292.38 kN,  R_AB = 155.57 kN]

 

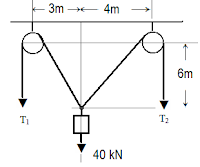
(2) A weight of 40 kN is suspended by two cables as shown in the figure. Find the tensions T₁ & T₂ in the cables. 

 3) On a square plate ABCD four forces are applied as shown in the figure. Find the resultant of the force system.

         

          4) A uniform bar AB length L and weight W lies in a plane with its ends resting on two smooth surfaces on OA and OB. Find angle θ for equilibrium of bar.

             [θ = 30° ]